The experimental method of the DeLLight project is as follows (see Figure 1). An ultra intense femtosecond laser pulse (2.5 J, 30 fs), named pump pulse, with a polarization s, is focused by an off-axis parabolic mirror (OAP) in the interaction area. A much weaker pulse (few tens of μJ), with a perpendicular polarization p, is sent into a Sagnac interferometer via a 50/50 beamsplitter (BS-2), generating two daughter pulses (Probe and Ref) that circulate in opposite directions around the interferometer. The interferometer is in a right-angled isosceles triangle configuration, formed by the beamsplitter and two mirrors (M1 and M2). The length of the longest interferometer arm between M1 and M2 is about 1 m. Both counterpropagating pulses are focused in the interaction area via two optical lenses (L1 and L2) of focal length f inserted in the interferometer between the two mirrors. One pulse (Probe) refers to the probe pulse that is
counterpropagating with respect to the pump, and a delay stage ensures the time coincidence of the arrival of this probe pulse with that of the pump pulse in the interaction area. The second pulse (Ref) is not in time coincidence and does not overlap with the pump. This pulse is therefore unaffected by the pump and refers to the reference pulse. The focus axis of the pump is transversally (vertically) shifted with respect to the focus axis of the probe (see Figure 2), thus engendering an impact parameter b so that the perturbation of the probe is asymmetric and the mean deflection is nonzero. In the absence of the pump, the two counterpropagating probe and reference pulses are phase shifted by π in the dark output of the interferometer, where a CCD camera measures the transverse position of the residual intensity profile. When the pump pulse is present and interacts with the probe pulse, the wave fronts of the probe are refracted by the induced vacuum index gradient, while those of the reference pulse are unaffected. After recollimation, the refracted probe pulse is transversally (vertically) shifted with respect to the unrefracted reference pulse by an average distance δy = δθ × f , where δθ is the average deflection angle of the refracted probe pulse. The interference of the probe and reference pulses in the output of the interferometer produces a transverse vertical displacement Δy of the barycenter of the residual intensity profile, which is measured by the CCD camera.
The advantage of this interferometry method is that the transverse shift Δy on the intensity profile in the dark output of the interferometer is amplified, as compared to the would-be shift δy when using a standard direct pointing method. The amplification factor A is inversely proportional to the square root of the extinction factor F of the interferometer (F = Iout/Iin where Iin is the intensity sent in the Sagnac interferometer and Iout is the intensity in the dark output of the interferometer).
With an energy E=2.5 J (delivered by the LASERIX facility at IJCLab), a minimum waist w0 = 5 μm, a focal length f = 50 cm, and an extinction factor F=10-5, as currently measured with our prototype, the expected signal is Δy = 0.015 nm.
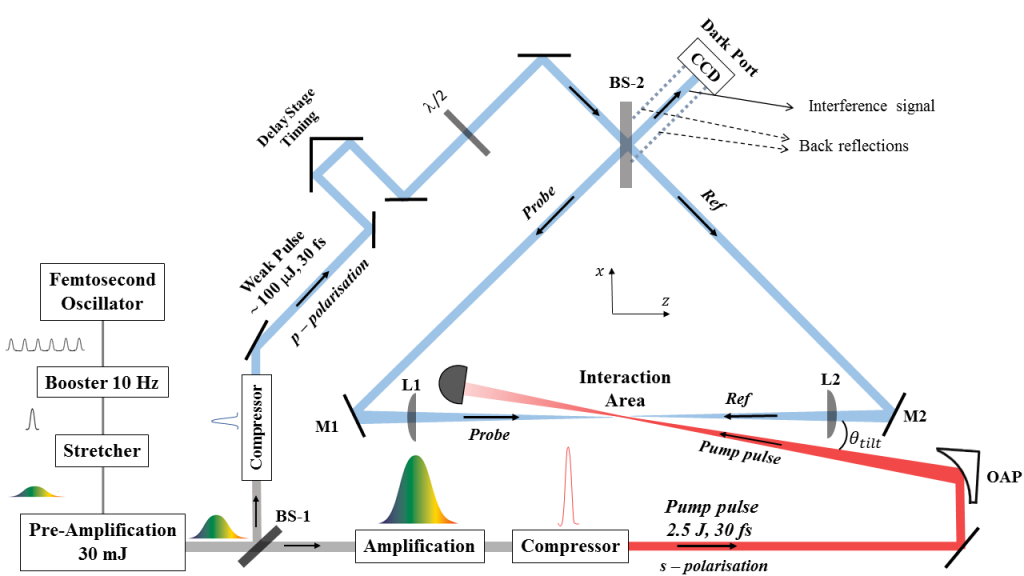
Figure 1: Schematic view of the DeLLight experimental setup
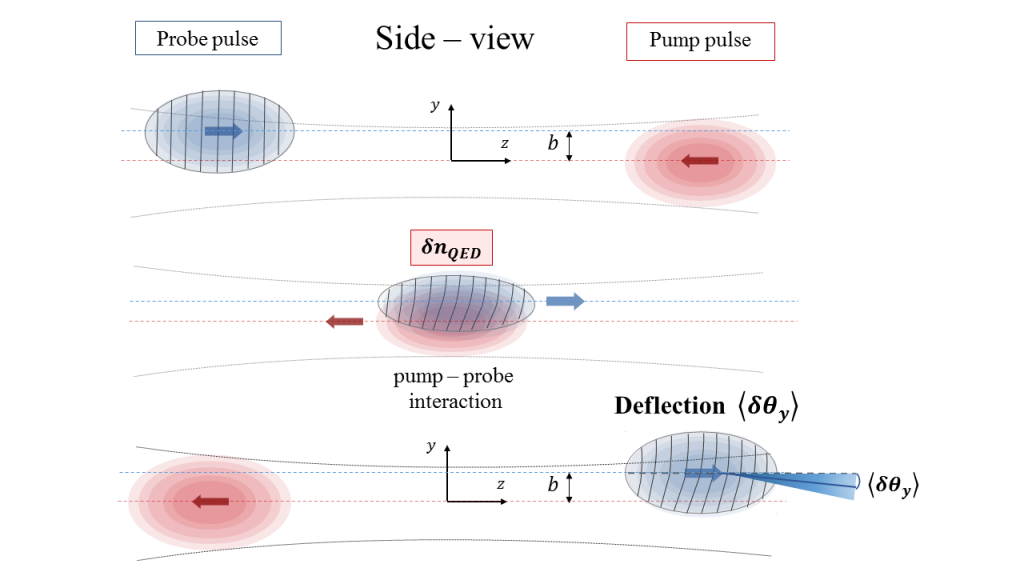
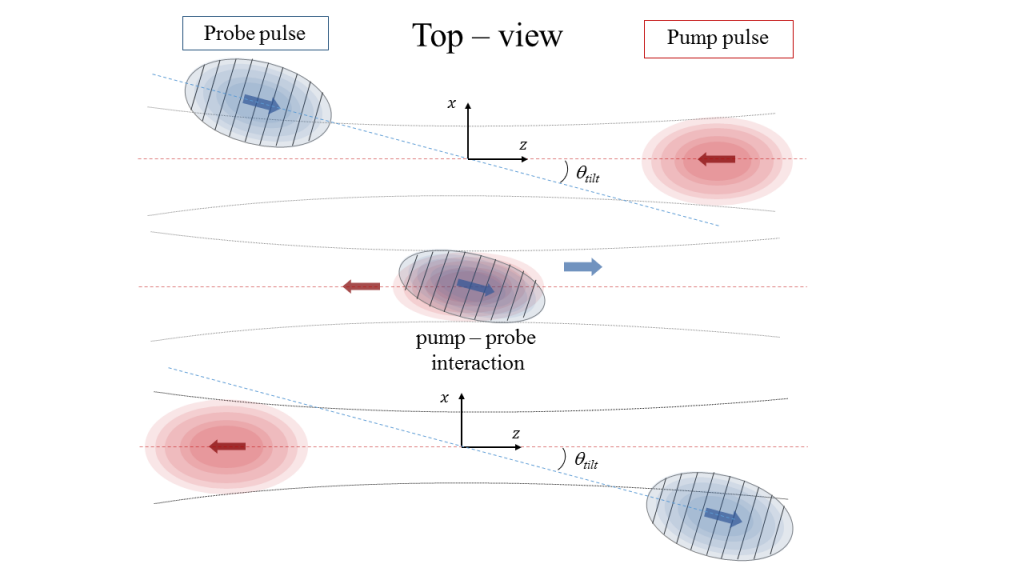
Figure 2 : Schematic view of the interaction between the probe pulse (in blue) and the counter propagating pump pulse (in red). The lines inside the probe pulse correspond to the wave fronts, which are gradually rotated by the vacuum index gradient induced by the pump. Side-view: The axis of the pump beam is vertically (y axis) shifted with respect to the axis of the probe beam, thus engendering an impact parameter b so that the perturbation of the probe is asymmetric and the mean deflection is nonzero along the vertical y axis. Top-view: In the horizontal plane (corresponding to the Sagnac interferometer x-z plane), the axis of the pump beam is tilted by an angle θtilt with respect
to the axis of the probe beam. The perturbation of the probe is symmetric and the mean deflection is zero along the horizontal x axis.
